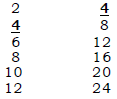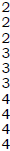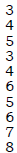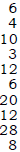Fractions, Ratios, Money, Decimals and Percent
What is the next fraction that we can divide the S-5 square into?
Students: (After trying for awhile) Eighths.
Teacher: Show me all the ways that you can find.
(illustration 11-5-8)
(Show the T-7, T-8 and R-1 shapes as eighths, with the halves and fourths still
visible.)
Teacher: I'll add what you have found to my list.
1 = 2/2 = 4/4 = 8/8
1/2 = 2/4 = 4/8
1/4 = 2/8
Teacher: Look at the numerators and the denominators of
all these fractions and see what
patterns you can find. Share with the rest of us any patterns that you see.
Our students saw these same fraction patterns when they folded paper in half and
then in half again.
Teacher: The next fraction that we can divide the S-5 square into is sixteenths.
Can you use the
patterns that you see to help you predict the numbers we will record before you
use your
blocks to find out?
In mathematics we look for patterns everywhere. Equivalence is a pattern we can
learn to see.
Lesson Six
| Purpose |
Learn to use equivalencies to find factors. |
| Summary |
Students use Unifix Cube sticks to generate lists
of
equivalencies which, combined with Start with, go bys, they
search for patterns to help them make sense of factors. |
| Materials |
Unifix Cubes, butcher paper, Start with, go by
chart, Power
Blocks. |
| Topic |
Unifix equivalencies are recorded and explored. |
| Topic |
Finding common denominators to use to add unlike
fractions.
Specific techniques taught depend on the patterns that the
students see and the sense they make out of their equivalency
experiences. |
Cube sticks...
Folded paper and Power Blocks give our students an opportunity to look for
patterns in equivalencies.
Unifix Cube sticks expand the opportunity.
Teacher: Make one cube stick eight cubes long.
(illustration 11-6-1)
(Unifix Cube stick eight cubes long.)
Teacher: How many sticks did I ask you to make?
Students: One.
Teacher: One is what we say it is. This time one is the cube stick that you just
made. How many
different fractions can you break your cube stick into? Remember, the rule for
fractions is
that every part must be of equal size. For your cube sticks, this means the way
you break
your cube stick up must give you little stacks of cubes all of equal size.
We'll do this first cube stick together, so I can show you what I mean.
(illustration 11-6-2)
(Show the ways to break the cube stick into fractions, with each way labeled. An
eight stick breaks into
halves and fourths and eighths.)
Teacher: I'll write the fractions we have found.
1/2
1/4
1/8
Everyone make a cube stick nine cubes long. One is now a cube stick nine cubes
long. How
many different fractions can you break this stick into? Remember, every part
must be of
equal size.
Show me all the ways that each of you can find.
(illustration 11-6-3)
(Unifix Cube stick nine cubes long. Show the ways to break the cube stick into
fractions, with each way
labeled. A nine stick breaks into thirds and ninths.)
1/3
1/9
Teacher: Now see what different fractions you can find
for a cube stick ten cubes long.
(illustration 11-6-4)
(Unifix Cube stick ten cubes long. Show the ways to break the cube stick into
fractions, with each way
labeled.)
1/2
1/5
1/10
Teacher: Now see what you can find for a cube stick of eleven cubes.
1/11
Longer sticks do not always mean there are more fractions to be found. A cube
stick of eleven cubes
makes elevenths and nothing more.
(illustration 11-6-5)
(Spaces numbered from one to 36 marked off on a big piece of butcher paper for
recording the fractions
for the cube sticks. The numbers are at the top of columns marked off to define
the writing space for the
fractions to be written beneath each number. The fraction numbers for eight,
nine, ten and eleven are
already written on the paper beneath their respective 8, 9, 10 and 11. Include
in the caption that as
much space is marked off as is available. Any number from 30 to 60 is a good
number with which to
work. More than 60 is nice if there is space. Also note in the caption that we
put this on butcher paper
and not on the chalkboard so we may save it more easily.)
Teacher: Up until now, I have been doing all the recording. Now it is your
turn to write the
fractions for the sticks.
You and your workmate can decide with which cube-stick lengths you wish to work.
For any
cube-stick length you choose to be one, write the fractions that you find in the
space beneath
its number on the paper at the front of the room.
Before you write your numbers on the paper, see if someone else has already
written fractions
there. If they have, then see if you agree with what they have written, and
write in only the
new fractions you have found.
Write large enough so that everyone can see what you have written, but not so
large that your
numbers go into the next column.
When you find all the fractions that you can for a cube stick, select another
cube stick and find
fractions for it.
Are there any questions? Then you may begin.
We may think our instructions are clear. We may even think that the absence of
questions from our
students is a measure of the power of our words to communicate. We know,
however, that the evidence
of the clarity of our words is in the work our students do. They will show us
soon enough if we need to
use a different set of words to make our meaning clear.
Patterns waiting to be seen...
The numbers that our students write are patterns waiting to be seen.
(illustration 11-6-6)
(Fractions and equivalencies beneath the number on the butcher paper.)
What kinds of questions might we ask for the numbers on the paper?
Is there a pattern for which cube sticks have halves as fractions?
Is there a pattern for the thirds and fourths and fifths?
Is there a pattern to the pattern?
Can you use the pattern to see any fractions that we might have forgotten to
include?
Can you use the pattern to tell what fractions might be made from cube sticks
whose lengths are not
written on the paper?
Which sticks have the most fractions?
Which have the fewest?
Why?
One pattern that our students are likely to see is that halves occur every
second stick, thirds occur
every third stick, fourths occur every fourth, fifths occur every fifth and so
on. Another observation is
that the sticks that break into the most fractions are not necessarily the
longest sticks. The twelve-cube
stick breaks into many more fractions than does the thirteen-cube stick. The
number of fractions that
can be made is not a function of a stick's length.
What happens, depends...
1/2 + 1/4 =
Teacher: Can you add one-half to one-fourth? If you can, can you prove the
answer that you find?
What happens now depends on what has happened before. What have our students
understood of
equivalencies? What patterns have they seen? Can they put their understanding to
use without being
told specifically what to do? Can they discover for themselves the patterns for
adding fractions with
denominators that are not the same? Will the patterns they discover serve them
as well for the next
problem that we pose?
Teacher: What is 1/6 plus 1/7?
Our students may have seen patterns in their cube sticks that will lead them
collectively to see how
they might add one-sixth to one-seventh. However, if our students need
assistance in making the
connections, we start with 1/2 + 1/4.
Teacher: Let's see if we can figure out what cube stick to use to add
one-half to one-fourth. What
is the shortest stick that will break into halves and fourths?
Is the chart for fractions that our students made still posted on the wall? If
not, there is another way to
calculate the shortest stick.
Teacher: Let's use Start with, go by, both to find the stick to use.
(Beginning Addition and
Subtraction, Lesson Two, page 000.) Take the denominators for each fraction
as the Start with,
go by numbers and see which number comes up first in both columns.
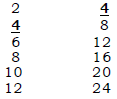
Teacher: Which number is the first to appear in both
columns?
Students: Four.
Teacher: Please make two cube sticks four cubes long. Each of these cube sticks
is one.
Show me one-half of the first stick and one-fourth of the second stick.
Now add the half to the fourth.
(illustration 11-6-7)
(Two cube sticks, four cubes long. Each stick is a different color. The first
stick broken in half. The
second stick broken into fourths. One of the halves and one of the fourths
separated out, with the half
added to the fourth.)
Teacher: What is one-half plus one-fourth?
Students: Three-fourths.
Our students may understand that the answer is three-fourths, or we may have to
teach them that each
cube for each cube stick in our example is a fourth. Three cubes snapped
together make three-fourths.
What happens depends on what our students need to know and on what they
understand.
1/4 + 2/3 =
Teacher: Now, let's see if we can figure out what cube stick to use to add
one-fourth to twothirds.
How do we find the shortest stick that breaks into fourths and thirds?
(illustration 11-6-8)
(Show all of the steps of the 1/4 + 2/3 problem worked out with cubes and
recorded in numbers. Use
cube sticks of two different colors. Also show the start with, go bys.)
Whether our students know that twelve is the common multiple for thirds and
fourths or they use Start
with, go bys to find out, depends on the patterns they have seen and the
connections they have made.
We watch to see if our students can solve problems on their own or if they need
us to guide them
through the steps.
What happens in any lesson always depends on what has gone before. Our students
show us when
they need our help and when they know enough to make connections for themselves.
Factors...
In Beginning Addition and Subtraction, Lesson Two, we asked our students what
patterns they could see
in the both numbers if they knew the two Start with, go by numbers. We saved
their Start with, go by
chart for a later time. That later time is now. The pattern is the same. If our
students could not see the
pattern then, we help them see it now.
Teacher: Factors are the numbers that we multiply together to get a bigger
number. Six is the
first number on our chart in both columns. What numbers multiply together to get
six?
Student: Two times three.
Teacher: Okay. Any other numbers?
Student: One times six.
Teacher: Any other numbers?
Student: Three times two.
Teacher: True, but we'll count 3 x 2 as the same as 2 x 3 and 6 x 1 as the same
as 1 x 6. The
factors of six are 1, 2, 3 and 6.
Any other numbers?
Student: No.
Teacher: Four is the next both number. What are all the different numbers that
multiply
together to get four?
Student: Four times one and two times two.
Teacher: So the factors are 1, 2, and 4.
Let's record what we are finding out. I won't record the ones, since one is a
factor for every whole
number.
| Start with |
Start with |
Both |
Both factors |
 |
 |
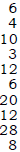 |
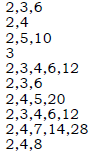 |
By adding factors to the chart, our students have more
information to use in their pattern search.
Teacher: What patterns can you see in the column of both factors on this
chart?
Student: All the numbers have themselves as a factor.
Student: Three has the fewest factors.
Student: Twelve and twenty-eight have the most.
Teacher: What patterns can you see that might help you know what the both
numbers would be if
you knew the two start-with numbers?
What was the answer that our students gave when we asked this same question in
Beginning Addition
and Subtraction, Lesson Two? What might be their answer now?
Student: The Start with, go by numbers are factors of the both numbers.
Teacher: Two and three are factors of six. But two and three are also factors of
twelve. Why do
you think six is the first both number for two and three and not twelve?
Student: Because you can divide two and three into six.
Teacher: But, you can divide two and three into twelve.
Student: But twelve is bigger. The both number is the smallest number both
start-with numbers
can divide into! Look! That's true for all the start-with numbers on the chart!
Teacher: That seems to be true for all the numbers on our chart so far. Let's
find some more startwith
and both numbers to see if the pattern holds up.
Factors are a pattern we can see in Start with, go bys or in dividing numbers or
in cubes. Factors are
also numbers to use for adding or subtracting fractions when the fractions do
not have denominators
that are the same. Can our students use the patterns they see now to help them
know the denominators
to use for equivalencies?
Problems...
When we teach a concept, how can we ensure the concept is understood? We use a
range of problems
to expose our students to the variety of possibilities that exist. Adding 1/4 to
1/2 is not the same as
adding 1/4 to 1/3. Adding 1/6 to 1/7 is different, still. We use materials and
situations in our class
and outside of school to create problems that have meaning for the students in
our room.
With Power Blocks and cubes:
(illustration 11-6-9)
(One Power Block S-5 square by itself. Next to it, an S-5 square made up of an
assortment of pieces.
List the specific pieces, so the illustration matches the numbers below.)
Teacher: The S-5 square is one. What are the fractional values of all the
other pieces I have used
to make the square?
1 = 1/2 + 1/4 + 1/8 + 2/16
The equation we have written is for Power Blocks. Can you prove to me that 1/2 +
1/4 + 1/8 +
2/16 = 1 using cubes?
With paper folding and cubes:
(illustration 11-6-10)
(A paper folded in half with 1/2 written on one half. The same paper folded in
half again with 1/4
written in one of the fourths created by the new fold. The 1/4 is NOT written on
any part of the section
already labeled 1/2. The same paper folded into thirds (meaning the smallest
section is now twelfths).
1/12 is written in each of the twelfth sections that were not previously labeled
1/2 or 1/4. The equation
1/2 + 1/4 + 3/12 = 1 is written beneath the paper foldings.)
Teacher: Can you prove that 1/2 + 1/4 + 3/12 = 1 with cubes?
From word problems that our students create:
Teacher: What is the answer to this problem?
1/4 + 1/2
Student: Three-fourths.
Teacher: Who would like to tell me a story to go with these numbers? Brenda.
Brenda: I ate a half a pizza, then I ate another fourth, so I ate three fourths
of the pizza.
Teacher: How much of the pizza would be left?
Brenda: One-fourth.
Teacher: Let's draw a picture to see if Brenda's story matches the numbers on
the board.
(illustration 11-6-11)
(Drawing of stick figure Brenda eating a half and then a fourth of a pizza.)
Teacher: Now, let's see what other kinds of adding and subtracting stories we
can invent. You
can use your imagination or you can see if you can think of problems that are
real.
The students write and draw. The teacher adds words to the spelling notebooks as
needed.
From questions we might ask:
Teacher: How many examples of things that are one-half of something can you
think of?